Notice that in f circ g , we want the function gleft ( x right) to be the input of the main function {fleft ( x right)}. Examples of solving.
Composite Functions Examples. Decompose a composite function into its component functions. Composition of functions given tables of values functions ( f ) and ( g ) are defined by their tables as follows
Chapter 3. Polynomial and Rational Functions. 3.4 Zeros of From ppt-online.org
F(x) =( x−1 x)3 f ( x) = ( x − 1 x) 3. A composite function can be evaluated from a graph. Introduction the composition of two functions g and f is the new function we get by performing f first, and then performing g.
Chapter 3. Polynomial and Rational Functions. 3.4 Zeros of
The following function composition examples can be used to fully understand the process used to obtain a function composition. It should look like this: Thus, f0(x) = 7x6;f0(g(x)) = 7(4x2 +1)6 and g0(x) = 8x: The composite of two functions f (x) and g (x) must abide by the domain restrictions of f (x) and g (x).
Source: venturebeat.com
Learn more about composition of functions here. Suppose we want to calculate how much it costs to heat a house on a particular day of the year. Do you know of a real world example where you�d combine two functions into a composite function? Given the function f(x) = 3x + 5 and g (x) = 2x3 2 x 3.find.
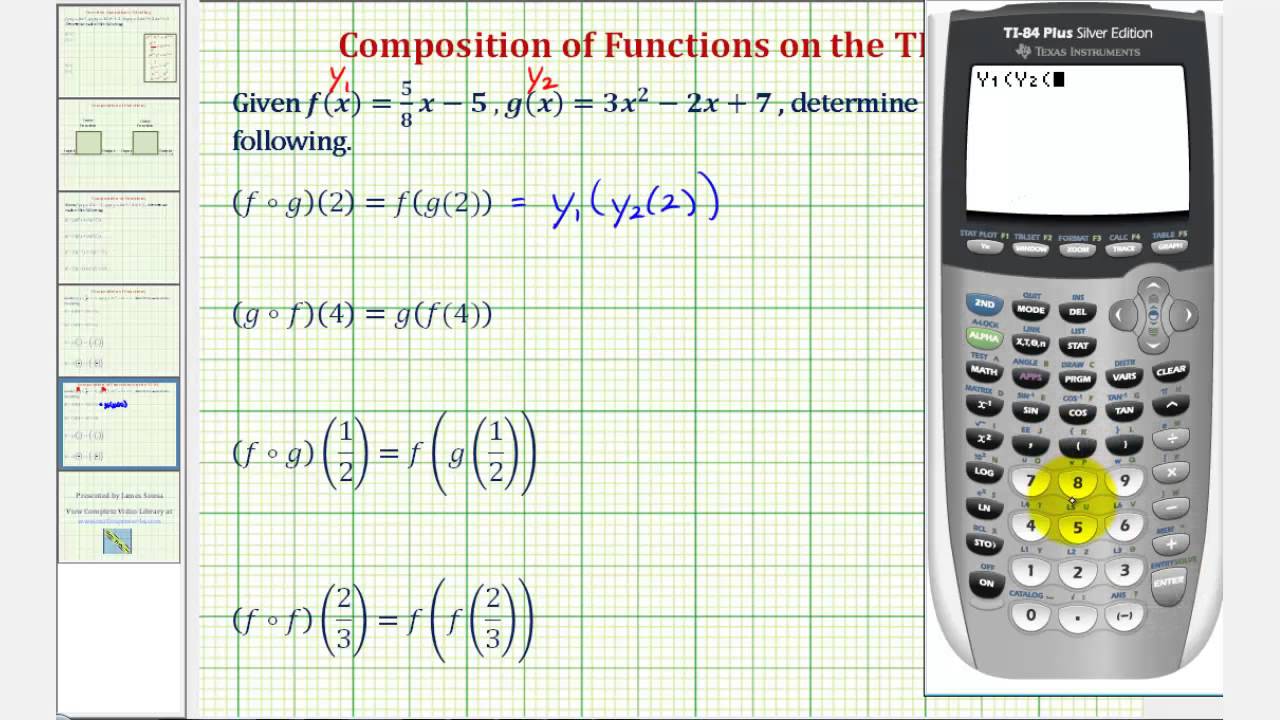Source: youtube.com
(g ∘ f) (x) =. Example 3.3.1 find the derivative of y = (4x2 + 1)7: It�s usually plug and chug where you take f (g (4) and run it through both functions. The composite of two functions f (x) and g (x) must abide by the domain restrictions of f (x) and g (x). Perform the indicated function composition:
Source: ppt-online.org
The cost to heat a house will depend on the average daily temperature, and in turn, the average daily temperature depends on the particular day of the year. Suppose that y = x p Thus, f0(x) = 7x6;f0(g(x)) = 7(4x2 +1)6 and g0(x) = 8x: The values coming out of a function are. The order of composition is important.
Source: youtube.com
Evaluating composite functions using graphs video khan academy examples of how to compose functions. First one’s life insurance cost goes up depending on the age of the buyer, that in turn is dependent on the current date. For example, if we let f be the function given by f(x) = x2 and let g be the function given by g(x).
Source: youtube.com
The above function can be broken down as a. One example of a composite function is the cost of life insurance as one ages. Given the functions, determine the value of each composite function. Given the functions, determine the value of each composite function. 8x 2 + 6 + 1.
Source: youtube.com
The above function can be broken down as a. This project was created with explain everything™ interactive whiteboard for ipad. This composite function is defined if (x ) is in the domain of ( g ) and ( g(x) ) is in the domain of ( f ). One example of a composite function is the cost of life insurance.
Source: ppt-online.org
This project was created with explain everything™ interactive whiteboard for ipad. G (f (x)) = 2 (4x 2 + 3) + 1. A composite function can be evaluated from a graph. The composite of two functions f (x) and g (x) must abide by the domain restrictions of f (x) and g (x). For example, if we let f be.
Source: ppt-online.org
The composite of two functions f (x) and g (x) must abide by the domain restrictions of f (x) and g (x). (g ∘ f) (x) =. It�s usually plug and chug where you take f (g (4) and run it through both functions. Examples of solving composite function problems are presented in this video. Example 3.3.1 find the derivative.
