If you now reverse the process with xstart = − x0 being compressed to xfinish = 0 and use the work done by the spring equation you will get +.
Equation For Work Done By Spring. Then click on the active text for the quantity you wish to calculate. The displacement x is measured from the undisturbed position of the spring (that is, x=0 when f=0).
PPT Work, Energy, and Power PowerPoint Presentation From slideserve.com
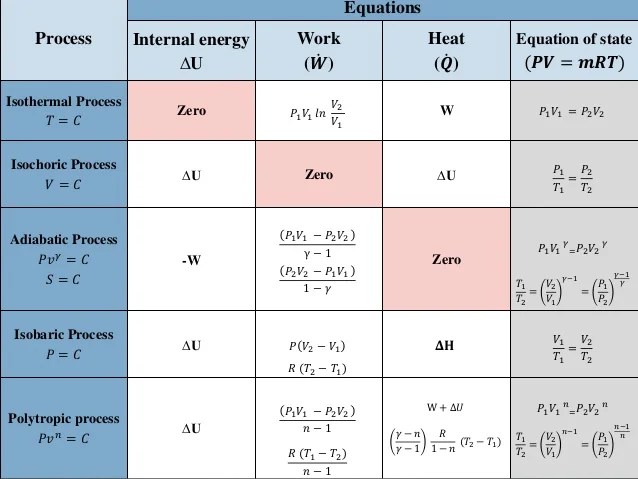
F=kx where k is the spring constant. Work = (force) (distance) w = ( f) ( x) if the force is constant, then the graph of force versus distance will be a rectangle and the area under the rectangle = the work done. W = (1/2) k x2.
PPT Work, Energy, and Power PowerPoint Presentation
$$ pe_{spring} = \frac{1}{2}k(\delta x)^2 $$ if i understand the question correctly, your $\delta x$ is the 30 centimeters, or 0.3 meters. The force is defined to be linearly increasing with the distance, x: F=kx where k is the spring constant. So, the first thing that we need to do is determine the spring constant for this spring.
Source: slideshare.net
The force f exerted by a spring is. The elastic potential energy stored can be calculated using the equation: F = k⋅ x f = k ⋅ x. When the spring is stretched by an external force, the work done by the spring, [w = \dfrac{1}{2}k{x^2}] where [k]=the force constant of the spring, [x]= the extended or stretched of the.
Source: slideshare.net
When a spring pulls something, or pushes something, over a distance x, it does work 2 work = 1/2 * k * x if a spring is compressed (or stretched) it stores energy equal to the work performed to compress (or stretch) it. The displacement of the spring from its. The work required to stretch or compress a spring. This.
Source: slideshare.net
Since the forcehas the form. If we continued to apply that force of n newtons to move the mass some distance, d meters, then he work done would be w = n*d joules. How much work did it take to stretch the spring to this point? This is stored workthat can be used to do work onsomething else bythis spring..
Source: youtube.com
We might call this spring potential energy. It is important to remember that the (x) in this formula is the distance the spring is stretched from its natural length and not the actual length of the spring. When the spring is stretched by an external force, the work done by the spring, [w = \dfrac{1}{2}k{x^2}] where [k]=the force constant of.
Source: slideserve.com
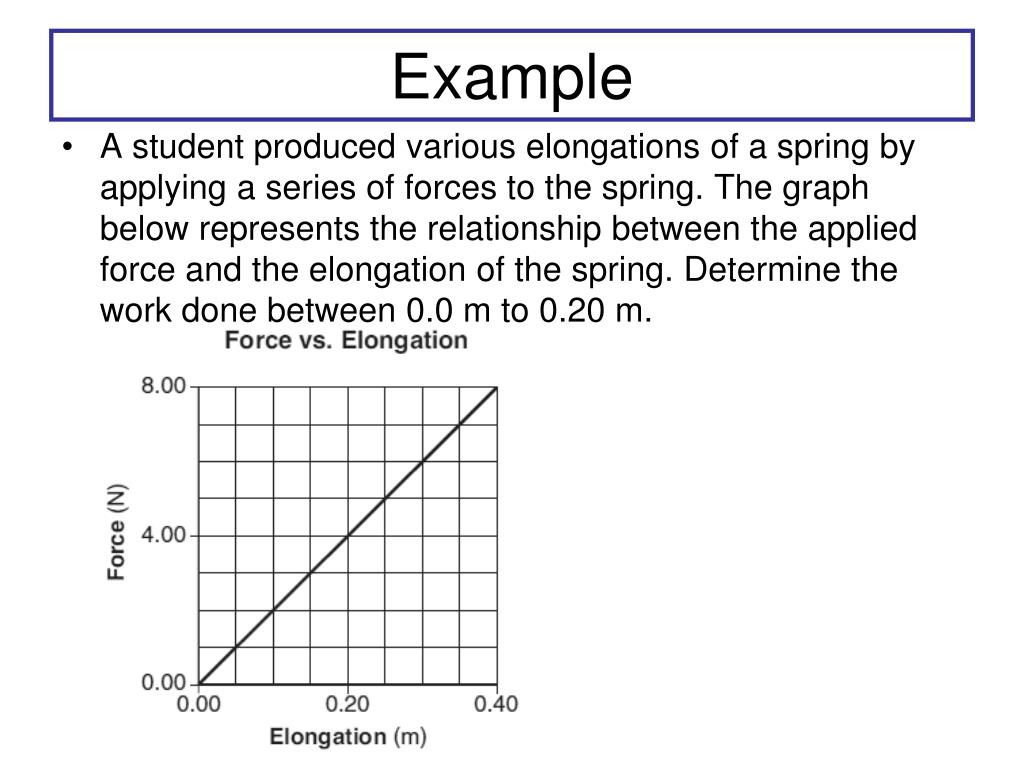
This work done is nothing but the elastic potential energy of the spring. You may enter data in any of the boxes. If a force f is considered that stretches the spring so that it displaces the equilibrium position by x. We can do that using the initial information. When a spring pulls something, or pushes something, over a distance.
Source: slideshare.net
F = k⋅ x f = k ⋅ x. Work = (force) (distance) w = ( f) ( x) if the force is constant, then the graph of force versus distance will be a rectangle and the area under the rectangle = the work done. Therefore, work = (1/2)kx 2 = (1/2)(100 n/m)(4 m) 2 = 800 joules example 2:.
Source: slideserve.com
Work = (force) (distance) w = ( f) ( x) if the force is constant, then the graph of force versus distance will be a rectangle and the area under the rectangle = the work done. The minus sign indicates that the spring force is always opposite in direction from the displacement of its free end. The constant k is.
