If p (xy) p ( x X2 + y2 = r2 x 2 + y 2 = r 2.
Equation Of A Circle Centered At The Origin. We could plug in x = r cos θ, y = sin θ to convert to polar coordinates, but In rectangular coordinates, the equation of this circle is:
How do you find the cartesian graph of r = 5sin(θ)? Socratic From socratic.org
The equation for a circle centered at the origin is: For a circle centered at the origin, this becomes the more familiar equation x2+y2=r2. A circle centered at the origin has a centre at (0, 0).
How do you find the cartesian graph of r = 5sin(θ)? Socratic
If it has a radius r, the equation is: So in general we can say that a circle centered at the origin, with radius r, is the locus of all points that satisfy the equations. Pin di worksheet if the squared terms have different coefficients the graph wont be a circle. Finally, which is the standard equation for a circle centered at the origin with radius?, the standard form for the equation of a circle with radius r ,.
Source: socratic.org
Write an equation of each circle described below. This is the equation for a circle centered at the origin. For a circle centered at the origin, this becomes the more familiar equation x2+y2=r2. (x − 0) 2 + (y − 0) 2 = r 2 x2 + y2 = r2. Axis of symmetry center circle (16 more) conic section directrix.
Source: youtube.com
The circle is centered at the origin, which means its center is the point (0, 0). If p (x;y) p ( x; Since the center of the circle is at the origin, i.e., at the point (0, 0), and its radius is r = 9, then the equation for this circle in standard form is found by substitution as follows:.
Source: metabunk.org
So in general we can say that a circle centered at the origin, with radius r, is the locus of all points that satisfy the equations. We have a new and improved read on this topic. Axis of symmetry center circle (16 more) conic section directrix ellipse foci focus hyperbola major axis minor axis nonlinear parabola radius systems tangent transverse.
Source: smart-answers.com
We draw a circumference as in the following diagram: This lesson covers finding the equation of and graphing circles centered at (h, k). $$x^2 + y^2 = r^2 $$. Click create assignment to assign this modality to your lms. Help us to improve mathematics monster.
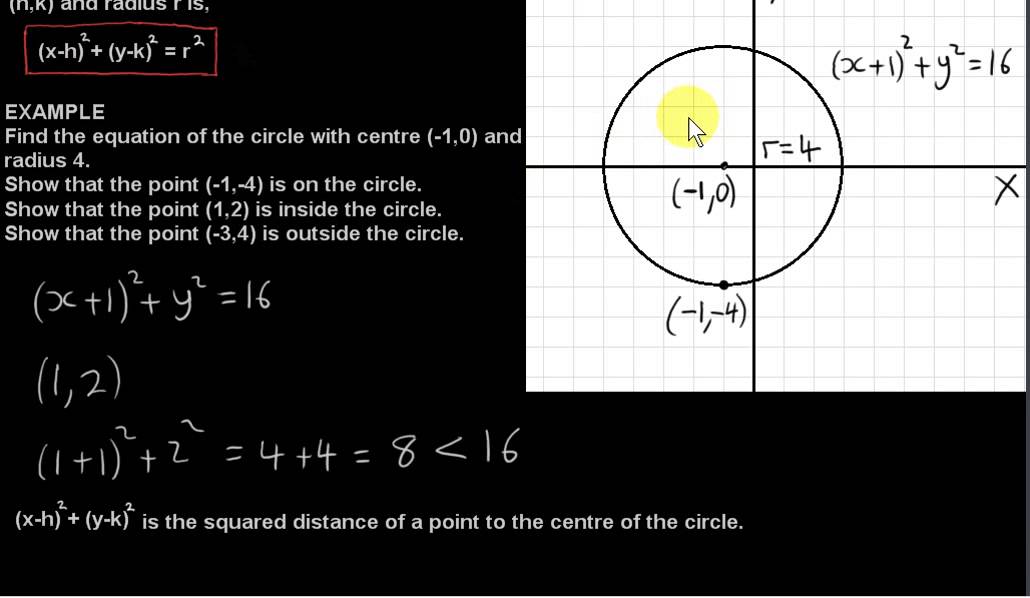Source: youtube.com
Given a circle with the center at the origin and passing through 4 3. In rectangular coordinates, the equation of this circle is: We have a new and improved read on this topic. For a circle centered at the origin, this becomes the more familiar equation x2+y2=r2. If p (x;y) p ( x;
Source: slideshare.net
Help us to improve mathematics monster. Using the completing the square technique converts the equation to an easier form. So in general we can say that a circle centered at the origin, with radius r, is the locus of all points that satisfy the equations. Since the center of the circle is at the origin, i.e., at the point (0,.
Source: youtube.com
The equation of a circle is a way to express the definition of a circle on the coordinate plane. $$x^2 + y^2 = r^2 $$. If p (x;y) p ( x; We could plug in x = r cos θ, y = sin θ to convert to polar coordinates, but (3, 2) on the circle.
Source: slideserve.com
Let�s substitute this into the equation. It also follows that any point not on the circle does not satisfy this pair of equations. If p (x;y) p ( x; About press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. Finally, which is the standard equation for.
